Greg Egan on Nostr: But they’re neither! The underlying symmetry can be seen in this tiling with ...
But they’re neither! The underlying symmetry can be seen in this tiling with triangles of angles π/2, π/8 & π/3. The “fundamental domain” of the Escher woodcut comes from 4 of these triangles.
The white curves are not hyperbolic lines. Rather, they are “hypercycles” or “equidistant curves”, which lie at a constant distance from true hyperbolic lines. The red curve is a true line, from which the white curve is equidistant.
https://en.wikipedia.org/wiki/Hypercycle_(geometry)
If that sounds strange, it’s because there’s nothing entirely analogous to it in Euclidean geometry: a curve equidistant from a straight line is just another straight line. And in spherical geometry, a curve equidistant from a great circle is a smaller circle.
A hypercycle is like a circle, in that it has a constant curvature along its length. But there is no point within the hyperbolic space itself from which it is equidistant.
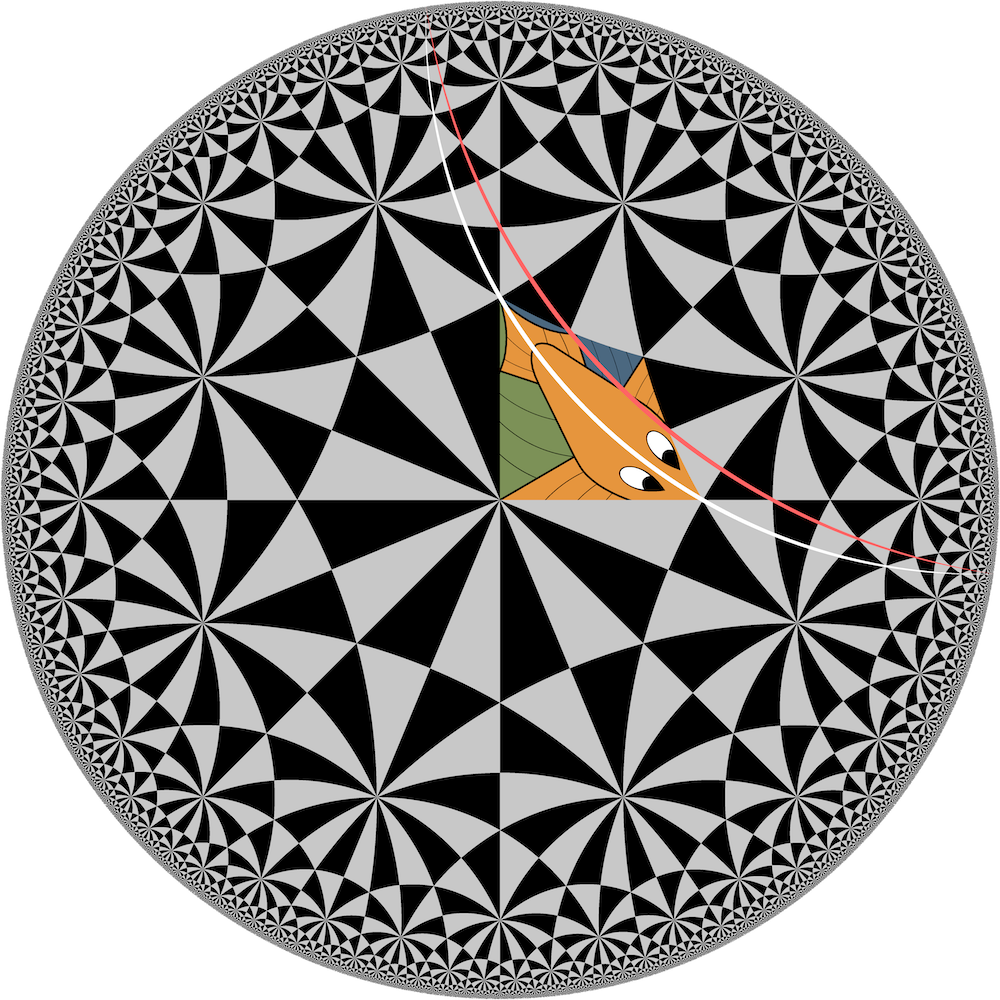
The white curves are not hyperbolic lines. Rather, they are “hypercycles” or “equidistant curves”, which lie at a constant distance from true hyperbolic lines. The red curve is a true line, from which the white curve is equidistant.
https://en.wikipedia.org/wiki/Hypercycle_(geometry)
If that sounds strange, it’s because there’s nothing entirely analogous to it in Euclidean geometry: a curve equidistant from a straight line is just another straight line. And in spherical geometry, a curve equidistant from a great circle is a smaller circle.
A hypercycle is like a circle, in that it has a constant curvature along its length. But there is no point within the hyperbolic space itself from which it is equidistant.
