John Carlos Baez on Nostr: "Hey, I discovered an amazing formula!" "Wow, that looks implausible. Did you prove ...
"Hey, I discovered an amazing formula!"
"Wow, that looks implausible. Did you prove it?"
"No, but I checked it to 15 decimal places!"
"You should have checked it to 20 decimal places."
It's great to make guesses in math. But it really does pay to prove them. A story like this actually happened:
https://mathoverflow.net/questions/251636/numerical-coincidence-why-is-sumxk2-sumxk1-22-for-x-0-8
In fact this "coincidence" is not a coincidence at all!
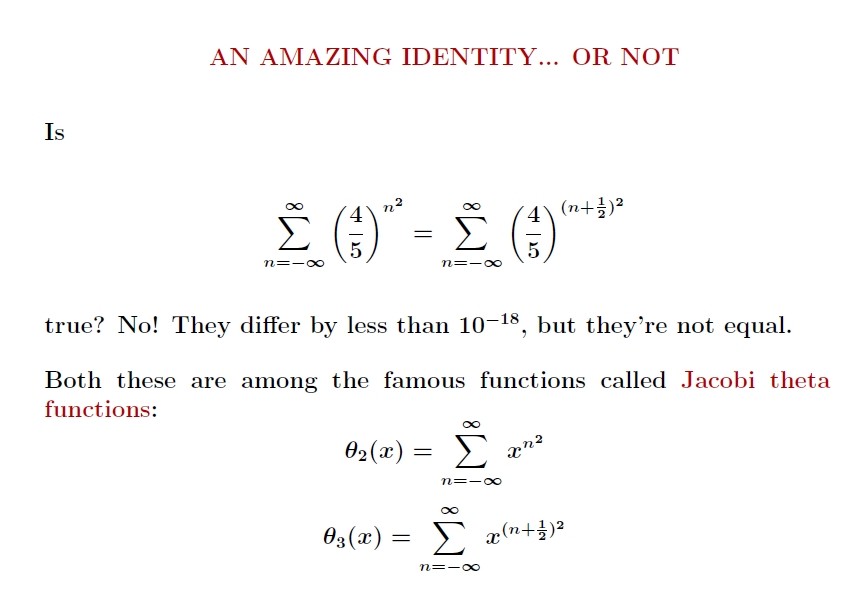
It's a fact about the Jacobi theta functions θ₂ and θ₃, which I define below. These functions are important in the study of elliptic curves. It's not very hard to show that as x gets bigger and approaches 1, we have θ₂(x) - θ₃(x) → 0. But fact it goes to zero very fast, so
|θ₂(4/5) - θ₃(4/5)| ≈ 9.3 × 10⁻¹⁹
We can go on with this game:
|θ₂(9/10) - θ₃(9/10)| ≈ 4.5 × 10⁻⁴⁰
and so on.
"Wow, that looks implausible. Did you prove it?"
"No, but I checked it to 15 decimal places!"
"You should have checked it to 20 decimal places."
It's great to make guesses in math. But it really does pay to prove them. A story like this actually happened:
https://mathoverflow.net/questions/251636/numerical-coincidence-why-is-sumxk2-sumxk1-22-for-x-0-8
In fact this "coincidence" is not a coincidence at all!
It's a fact about the Jacobi theta functions θ₂ and θ₃, which I define below. These functions are important in the study of elliptic curves. It's not very hard to show that as x gets bigger and approaches 1, we have θ₂(x) - θ₃(x) → 0. But fact it goes to zero very fast, so
|θ₂(4/5) - θ₃(4/5)| ≈ 9.3 × 10⁻¹⁹
We can go on with this game:
|θ₂(9/10) - θ₃(9/10)| ≈ 4.5 × 10⁻⁴⁰
and so on.
